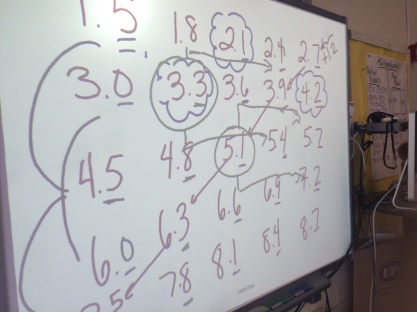Category Archives: Decimals

5th Grade: Decimal Place Value

There are some standards I think we do such a great job developing in early elementary, but never revisit explicitly when students learn about different numbers such as fractions and decimals. I blogged about this in reference to even and odd numbers last year, but this past week I have found another:

Screen Shot 2017-03-12 at 4.15.11 PM.png

Early elementary spends SO much time building understanding of the relationships between 1’s, 10’s and 100’s, but I don’t think we do this standard justice as students build their understandings of fractions and decimals.

Leigh’s 5th grade math class just started their work with decimals. To help students make connections to what they learned last year, she and I went back and brushed up on where the students should be in terms of the 4th grade CCSS. It is always so interesting to me how the CCSS authors chose to put decimals in the NF strand in 4th grade because students are learning decimals are just another way to write a fraction with a denominator of 10 or 100. Building on that understanding, the decimal work then moves to the NBT strand in 5th grade as students begin operating with them. Those are the little, thoughtful details in the standards that I really appreciate.

The first lesson or two of the unit, Leigh picked up where the students left off in the curriculum last year – shading 10 by 10 grids in a game called Fill Two. In this game, students only work with tenths and hundredths so in subsequent lessons she introduced thousandths on the same grid, with each small square now partitioned into 10 smaller pieces.

To be confident of the path we were on with decimals, Leigh and I met to revisit the CCSS. We both felt the students were doing 5.NBT.A.1 in a conceptual way, but we were never really making the understanding explicit with students. Since there wasn’t a great place in the curriculum for this, we went to Illustrative Mathematics, found this task and built a lesson around it.

We opened the lesson with this Which One Doesn’t Belong? to see how students related the representations, in particular, how they talked about the picture since the task had something similar.

Screen Shot 2017-03-12 at 4.28.58 PM.png

They came up with some really interesting comparisons and everyone saw the picture of base 10 blocks as 0.12 and “not even close to 1,” which the others were. This is where I think the lack of attention we give the standard above is really apparent. I think students learn each small cube is 1/100, so each skinny tower is 1/10, but are never pushed to think about what other values they could represent…how quickly they forget K-2:).

We then did a Notice/Wonder with the image from the task before they jumped in to work on the task prompt.

They did a great job trying to get into Jossie’s head, so we let those ideas sit there as we gave them 3 minutes of individual work time to begin the full task. After time to work with a partner, we came back together to discuss Jossie’s reasoning and the different values the picture could represent.

As we anticipated, many of the students said the Jossie got the tenths and hundredths pieces confused.

So polite in her disagreement, I love it!

Then, one student explained her reasoning below:

After her explanation, I asked the rest of the class what they thought about how tens and hundreds are related versus tenths and hundredths. It was a great discussion of how tens build to hundreds but hundredths build to tenths. My summary here doesn’t do a bit of justice to how the students were talking about the math.

I collected their possible values for the picture and recorded them on the board.

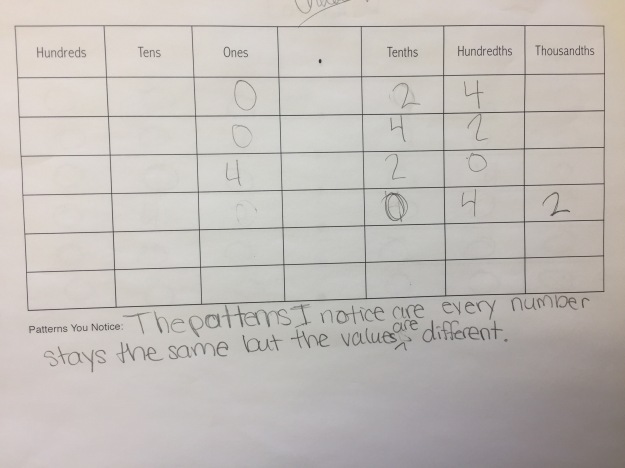
Students took these values and put them into a place value chart to look for patterns:

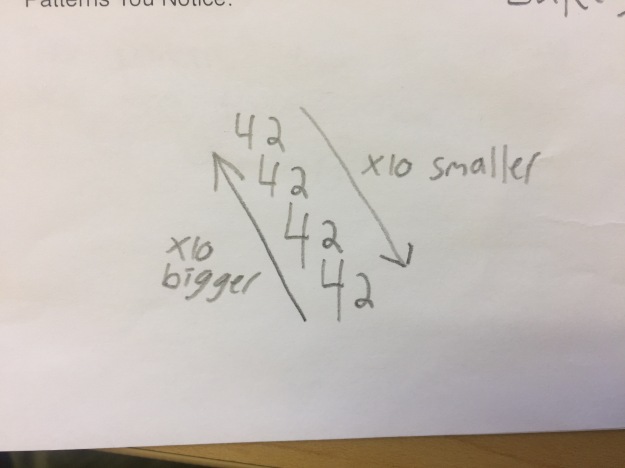
Some noticed the 4 and 2 were constant in each number and moved around:

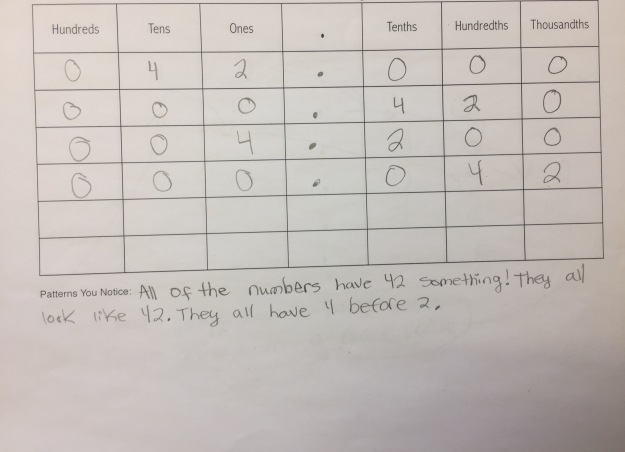
Some students recognized those movements as multiplying or dividing by 10’s:

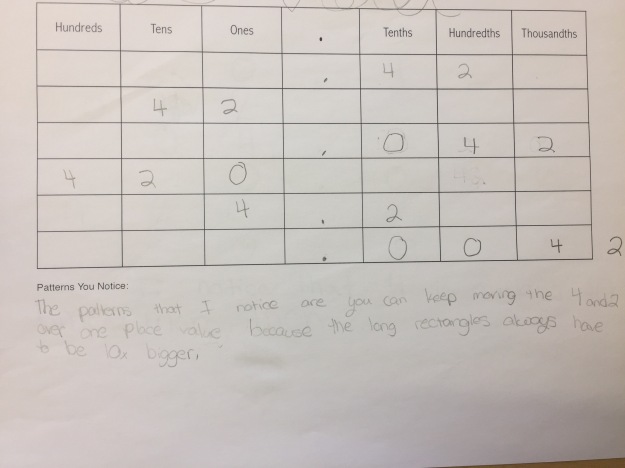
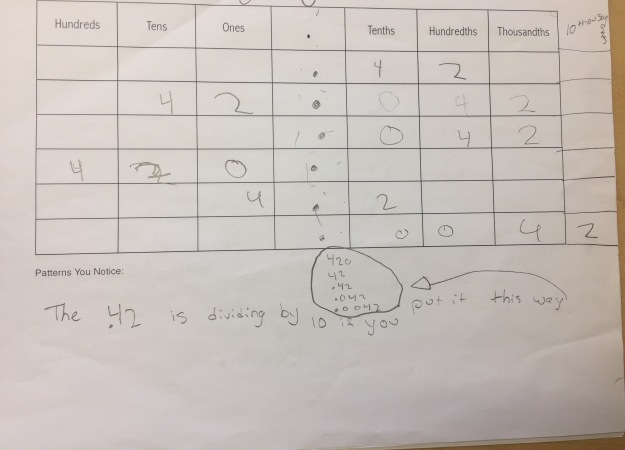
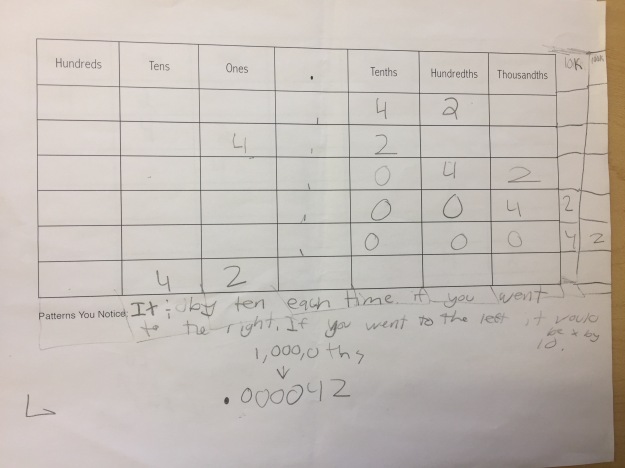
These last two examples are the ones that really seem to get at the standard and something we want to connect the previous examples to build toward:

Decimal Addition Card Sort

1 Reply

When solving problems in Number Talks, the strategies, en route to the solution, are the focus of the discussion. However, not all problems posed during a Number Talk are created equal or solved the same way every time by every student. While I know the majority of students use a particular strategy for one reason or another, whether it be because of the numbers involved or maybe it is the only strategy they are comfortable using, I like to take time and make these choices explicit. I want the students to think about the numbers before just computing and become more metacognitive about their actions.

Last week, the 5th grade teachers and I planned for a card sort to get at just this. The students have been adding decimals and using some great strategies, but we really wanted to hear about the choices they were making. With the help of the Making Number Talks Matter book, we chose problem types for students to think relationally between whole number and decimal operations. While there are no right or wrong answers, these are the problems we chose with strategies we thought went along with each. The expectation was not to have the students solving it the way we had listed, but to hear, and have other students hear the choices being made.

Screen Shot 2016-03-07 at 3.05.28 PM.png

We gave partners the cards, they sorted, and named the categories whatever they wanted:

While the card sort conversations were really interesting, the class discussion afterwards was amazing! There were so much questioning of one another about how one strategy is any different than another. For example, some groups used rounding for a problem that another group used compensation and another grouped called it using friendly numbers…so groups had the same problem in three differently-named categories. Again, the category was not important, but more the fact they were actually thinking about the numbers they were given.

The other 5th grade teacher and I are planning to do the same activity with multiplication when they get there! Excited!

Fraction & Decimal Number Lines

4 Replies

Recently, our 3rd, 4th, and 5th grade teachers had the opportunity to chat math for 2 hours during a Learning Lab held on a professional development day. It was the first time we had done a vertical lab and it felt like perfect timing as 3rd and 4th grade would soon be starting their fraction unit and 5th would be entering their decimal unit. Prior to the meeting, we read the NCTM article, “Identify Fractions and Decimals on a Number Line” by Meghan Shaughnessy, so we started the meeting discussing ideas in the article. We then jumped into playing around with clothesline number lines and double number lines, discussing what they could look like at each grade level based on where students are in the fractional thinking.

I have co-taught number line lessons in both 5th grade and Kindergarten this year, but both were a bit different in not only number, but organization. In 5th grade we used one clothesline with the whole class, while in Kindergarten we used tape on the floor and students worked in small groups. Leigh, 5th grade teacher, was interested in trying the small group number lines on the floor. As we planned the lesson, the one thing we thought would be difficult about having small groups is getting around to each group to hear their conversations, especially when we were planning cards purposefully to address misconceptions and misunderstandings. However, knowing we would have the two of us circulating, as well as two 3rd grade teachers who wanted to see the lesson (yeah!), we knew we had plenty of eyes and ears around the room to hear the math conversations.

During the lesson, in groups of three, students placed 24 cards on their number line. There were two sets of cards, so after placing all of their cards, each group visited a number line with a different set of cards to discuss. Instead of boring you with all of the number choices we made, here are a few of the choices in cards and the reason(s) we chose them:

1/3 and .3: Students often think these two are equivalent so before the decimal unit we were curious to see how they were thinking around that idea and how they used what they knew about fractions or percents to reason about it.

0.3, 0.33, 0.333, 1/3: The 1/3 and .333 were there to think about equivalency, while the others were there to think about what is the same in each and how much more each decimal has to make it larger. Which you can see caused some confusion here:

2.01 and 2.08: We were curious about the distance they put between these two cards.

1 6/9 and 1.6: We wanted to see how students compared fractions and decimals when they can’t easily convert 6/9 into a decimal. Then, if they began thinking 6/9 and 6/10, how would they decide on the larger fraction and then how much distance do they put between them?

The group below practically had them on top of one another:

While this group had a bit of a space between them:

2.8 and 2.80: Tenths and hundredths equivalency. They all seemed to handle this with ease.

.005 and 1/100: Curious to see the placement in relation to the other numbers. This 1/100 is close to 0 but I wonder about it in relation to the .2. Definitely a conversation worth having!

2.8 and 2 7/8: To see how they compared the 7/8 to the 8/10.

After they visited other number lines, they had a chance to meet with that group and discuss card placements they agreed with and placements they did not. Groups then made adjustments accordingly…

Here was a group’s completed number line and my first stab at panoramic on my phone!

The journal entry we left them with was, “Which cards were the most difficult to place on the number line? Why?” Many were just as we suspected.

The conversation as I walked back over to the other building with the 3rd grade teachers was, what does this look like in 3rd grade? Could we use array images to place on the line instead of the fractions? Could the pictures include over 1 whole? What whole numbers would we use? Do we play with equivalent pictures with different partitioning? Being mindful of the students’ second grade fraction exposure, below, we are planning on trying out something very soon! I am thinking the cards like these on Illustrative, with the pictures but no fraction names at this point.

CCSS.MATH.CONTENT.2.G.A.2
Partition a rectangle into rows and columns of same-size squares and count to find the total number of them.

CCSS.MATH.CONTENT.2.G.A.3
Partition circles and rectangles into two, three, or four equal shares, describe the shares using the words halves, thirds, half of, a third of, etc., and describe the whole as two halves, three thirds, four fourths. Recognize that equal shares of identical wholes need not have the same shape.

…and then could the journal could be, “Can you name any of the fractions on your number line? How do you know?”…or something like that!

Remainders: Division & The School Year

Creating Contexts for Decimal Operations

1 Reply

Sometimes I have students engaging in math within a context, however at other times, we just explore some beautiful patterns we see as we play around with numbers. I see a value and need for students to experience both. This week was one of those “number weeks” and it was so much fun!

Over the past few weeks, we have been working on decimal multiplication. If you want to see the student experiences prior to this lesson, they are all over my recent blog posts….it is has been decimal overload lately:) After sharing strategies and connecting representations in this lesson, I was curious how students thought about this problem in a context because up to this point, I had not given them one for thinking about a decimal less than one times a decimal less than one.After they wrote their problem, I asked them to tell me what they were thinking about as they were deciding on the context.

I anticipated that many would refer back to what they know about taking a fraction less than 1 of a fraction less than 1, like in this example…

I love how this one said she knew she “had to start with .4” That shows the order of the numbers in the problem create a context for her. It mattered to her, taking .6 of the .4.

This student went with two different contexts and again saying that he started with the .4. This must be something we have chatted about quite a bit about because it showed up multiple times. I loved how this student said he thought about an area model in creation of his problems.

This student was great in listing all of things he was thinking about as he thought about a context..

I had students who attempted to create a “groups of” context. I don’t know if I ever realized how difficult this and how much I, as an adult, need to be able to create a visual in my mind of what is happening in a problem to make sense of it. Here is one example (not the sweetest context but she thought the Mary HAD a little lamb was clever…) She worked a bit yesterday to show what the representation would be, but kept running into problems with cutting into “.6 pieces.”

And then I have these two that had my brain reeling for a bit, for many reasons. First, does the context work with this problem? Secondly, I knew it sounded like it should work, but when I tried to make sense of it, I couldn’t create a visual. Also, as I read them, I thought I knew where it was going and the question I would pose, but it wasn’t the way they saw it ending. I asked them to create an Educreations about their problem so I could check out their thinking around the context.

Yes, Rick Astly. But the question at the end, compared to the total time Never Gonna Give You Up, threw me a bit, not where I was going with it….

His Explanation: https://www.educreations.com/lesson/embed/31398809/?ref=embed

The second one tried it out, and wasn’t so sure of his question after messing with it. The wording “.6 as small” was making me think. I was trying to make sense of that wording, do we ever say six tenths times as small? Then does his question referring back to the .4 make sense?

His Explanation:https://www.educreations.com/lesson/embed/31402039/?ref=embed

Definitely a lot for me to think about this week too! I have some amazing work with them connecting representations to write up later…they are just such great thinkers!

-Kristin

Multiplying Decimals Less Than 1 Whole

1 Reply

Apologize, not much time to write, but today was so cool I had to share! I am in the midst of using this work to better plan for tomorrow.

Short version: After our predictions yesterday, I posed 0.4 x 0.2 (I changed the problem to 0.6 x 0.4 for my second class) and asked the students to individually jot down what they thought the answer is. I was looking to see how they intuitively thought about the problem. As expected, I saw 0.8 and 0.08 (2.4 and .24) as I walked around. I wrote both answers on the board, asked them to write their reasoning in their journals and then we shared as a class. No telling which was right or wrong, just sharing and listening.

Some great thinking and critiquing of each others’ reasoning ensued and then I sent them off to come to a consensus as a table and create a poster of how they thought about it!

Now, where to go with this work? They could just look at strategies, but I want them to think deeper about the meaning of the problem. After chatting with my colleague Faith tonight, who is coming to observe tomorrow, we are going to have the students walk around to the other group posters and talk about what they saw on the other posters that changed the way they thought about the problem.

From there, I really wanted them to think about a context for this problem and Faith suggested also thinking about what happens are you begin to adjust the numbers and why….really thinking about the reasonableness of answers. What happens when one factor increases? What happens when one factor goes over a whole? What happens if the factors go into the hundredths? Does the product increase or decrease? Why?

So many fun convos to be had tomorrow!

-Kristin

Making Decimal Predictions

3 Replies

Over the past weeks, I have done a lot of blogging about our work with decimal multiplication. All of this work has been focused around contexts that involve multiplication of a whole number by decimals both greater than and less than one. The students have very flexibly moved into using whole number strategies in order to multiply decimals during our number talks. Today I asked them to think about how whole numbers multiplication is similar or different from multiplication involving decimals. I was hoping to hear the relationship between the factors and the product and they did not disappoint. These are the findings from my two math classes…

I asked them to prove that a decimal greater than 1 times a whole number will have a product that is greater than both factors OR if a whole number, less than one, times a whole number will have a product that is less than one factor but greater than the other.

We shared out and ended the class predicting what they think would happen when we multiply two numbers that are less than one. This is where I saw an interesting difference in the way students thought about the problem. Some focused on the numbers and what it means in an “of” sense, while others connected to what happens with the multiplication process.

This makes for such an interesting conversation tomorrow! Excited to see the fractions come out and for students to revisit their predictions! This is the work tomorrow from last year’s experience: https://mathmindsblog.wordpress.com/2014/07/25/unanticipated-student-work-always-a-fun-reflection/

-Kristin

Investigating Patterns

2 Replies

Due to ELA testing, I luck out with an extra 45 minutes of math time twice this week, and today was one!! I wanted my students to revisit the choral count we did on Monday and look deeper into the patterns they noticed. To extend that thinking, I wanted them to make some predictions about decimals that may or may not show up if we continued counting by 0.3 (Thanks so much Elham for the suggestion:)!

We revisited the count and the noticings…

I then wrote some decimals on the board, shown inside the rectangles (kinda) in the first picture above. I asked them to try and use the patterns they discovered to decide if the decimals would show up if we kept counting by 0.3. I was sure to choose a range of options so everyone had an entry into the investigation and focused on the patterns we had discussed. I loved the way they explored their patterns and it completely intrigued me the manner in which they do so.

Some explored by multiples of 3 by looking at wholes and then tenths…

Some used the patterns that involved just one place value but did not look at the decimal as a number…

This group looked at the decimal as a number and chose one pattern they know would work for any number. They broke each decimal into partial quotients to see if each part was divisible by 3…

Other groups used a variety of patterns, noticing that some would work nicely for certain decimals and not others…

The next two especially caught my attention because I had not anticipated the connections being made (I ADORE the way they think:)..

Let’s look at the first one…He saw the “switching the digits around and the other decimal always shows up” pattern working every time and decided to examine the why. His approach was so interesting. He decided to look at the missing addend between the number and its “switch” each time. He noticed the missing addend was always a multiple of 0.9. He then started to look at the relationship between the original numbers and their missing addend. For example (and I so wish you could hear his thinking on this) the missing addend from 1.2 and its switch was 0.9 and the missing addend from 5.7 and its switch was 1.8, so what is the relationship between 1.2 and 5.7 that explains why the missing addend doubles? My curiosity is..what makes that be the next step for some students while others just notice it the missing addend is a multiple of 0.9 and are content. Loved this moment today because I got such insight into how students look at different pieces of a “puzzle” and choose to explore different relationships.

This one was so funn…

She noticed that any two numbers in her list (table), added together, had a sum that also appeared in the table or would appear, if extended. I asked her how she knew that and she showed me a few examples. “Ok, but why?” She thought for a while and then said, “Okay, it is kind of like the even plus and odd number will always give you an even number.” I could tell she was starting to make sense of the structure of numbers but having such a struggle in explaining it. To her, it seemed to just make sense and I think (hard not to make assumptions) that she was thinking about that 0.3 being a factor of both so duh, it just is.

She came back up, an hour later (she kept working on it when she left me:), and said she had it…”it is like DNA.” Ok, now I am intrigued. She explained it to me and I asked her if she could write that down for me because I thought it was so cool…

It seems like a stretch and I am still thinking about the connections, but I am stuck on the piece in which she says, ” …may look different but act similar…or act different but look similar….”

How many connections to factors and products, addends and sum and such ring true in this statement?? I love when they leave me with something to think about!!!

Another great day in math!

-Kristin

Connecting Whole Number Operations to Decimal Operations

2 Replies

I planned today’s number talk to draw out a variety of strategies for students to reflect on as they worked through their decimal work today. I used this series of problems:

4 x 18, 12 x 18, 39 x 18

After a variety of strategies such as partial products, area model, double/half, triple/third, friendly numbers, and adjusting a factor and product I paused when I posed the final problem and asked them to estimate. Thumbs went up right away and I go predominately two answers, 800 (from 40 x 20) and 720 (from 4 x 18 x 10). We discussed if it was going to be more or less than the actual answer and then we finished with a student subtracting 18 from 720 and arriving at 702.

While they were still on the carpet, I told them to be thinking of all of these strategies as they were going through their work today because we would be reflecting on them later. I posed the following problem and asked them to think about an equation and answer, “Bob is running 7 miles each day for two days, how many miles did he run.” I got 7 x 2 = 14 and then 2 x 7 = 14 because “it is two groups of 7 miles.” It was nice when a student said the commutative property makes that not matter for the answer. So I followed with, “What would it look like if he ran 0.7 mile each day? More or less than 14?” They said less because it is much smaller and we wrote 2 x 0.7 = 1.4.

They went back to their tables and I asked them to think about how we could represent these two equations on a number line. My thought was that it would give them a visual of the size (magnitude) of the jump and help in determining reasonableness. Eh, mistake on my part…I forced that number line on them and, while it was fairly easy for most, some really struggled. The upside was, it was a great formative assessment for me to see how students approach number lines (many like putting the 1/2 in the middle, yet had 14 on the end and were confused). We used number lines a lot in our fraction unit and definitely went past a whole on them, however I guess I did not really make that connection to fraction multiplication on a number line. Mental note for future work:)

After we had our number lines up on board and talked about determining reasonableness based on the factors. I posed this, “Let’s say Bob ran 2.8 miles a day for 8 days. What would be a reasonable estimate for his total miles.” They talked at tables, came back with 24, 20-24, and 17. We talked about the actual being more or less than each estimate.
Fabulous, now I want you to show how you could find the actual answer as many ways as you can.”

They went around to the different tables and talked about which strategies they had that were the same, one they maybe had not thought about and then which one they connected most with. After they finished walking around, I had them reflect on any of those questions in their journals…

Aww….”a bad number you can round to a happy number.” 🙂

I love this student picked this strategy up from another group!

“The distribution property”:)

Can you tell they did not take to that number line at all? Not one number line. I also anticipated some fraction work, but they were really working with the decimal in connecting with whole number multiplication. It was a really fun day of math!

-Kristin

Choral Counting – Decimals

10 Replies

The first day back to school after NCSM/NCTM is definitely an exciting one! I was excited to see my students, hear and see them doing math again, and incorporate the amazing things I learned at the conference with them. It is always great when I can go to a session, regardless of the grade level focus, and be curious how my students would engage in the activity. For example, I went to an amazing session on counting by Kassia (kassiaowedekind), Elham (@ekazemi) and Allison (@allisonhintz124). While the session focused on whole numbers, I began thinking about how I could take this same practice of Choral Counting and use it in my classroom. I have to admit, while my first thought was what my students would think about during this activity, I also had my own curiosities in the teacher organization of the work. Does writing them horizontally vs vertically bring out different noticings or patterns? or How does how many I put in each row or column affect their thinking about it?

Luckily, we are currently working on multiplication of decimals and I thought this would fit in just perfectly. I did some brainstorming and decided for my first class we were going to choral count by 0.3, record horizontally and vertically and have 1/2 of the class focus on the horizontal while the other 1/2 focused on the vertical. I was curious to see if they saw different patterns emerge. I started at 1.5 because I wanted a number that would hit a whole a couple times in our round but not make the “10” of them makes a whole number so obvious.

I did find that many of the same patterns emerged, however it definitely looked more intuitive for the students to look for patterns in the direction they had recorded.

Here are a few students who used the vertical recording…

You can see this student first noticed the 1.5 going up and down each column. She then noticed a diagonal pattern and could place the 9 where it would go had we continued.

This student started with thinking about them as whole number by multiplying them by 10. I love the last noticing because it makes such a beautiful connection to his first statement. When I asked him to clarify his thinking he did stipulate that you had to start at 3 for that to be true.

There is a beautiful statement in here that says she knew 0.3 is 10% of 3 because between each whole number there are ten 0.3’s. Lovely.

Here are some examples of horizontal, again, many of the same patterns…

This one was not so much focused on the patterns of numbers increasing or decreasing, but instead found that if you switched the whole number and tenths, the number would also be hit by a multiple of 0.3. Interesting to figure out why that works and when that doesn’t work. They left REALLY excited to keep working on this one. How much do I love the “I thought of this!” next to it!

I asked one student, who seemed content with his noticings before they shared as a table if he could think of any equations that matched the number patterns he saw while he waited.

I asked him where he saw the last one in the numbers and had him record it in Educreations: https://www.educreations.com/lesson/view/multiplication-decimals/31049585/

When the second class came in, I decided to switch up the number in each row to five (thanks Elham for that suggestion) to see if differences came out. Here was our board:

i definitely like the 5 in each row better than the 6, a lot more patterns emerged, quickly. It pretty much screamed patterns! We shared them all and I asked each table to pick one they wanted to explore deeper and figure out why it was happening.

This student said, “If you pick any number, go up and then over two the tenths digit will be one more than the starting number. It also works if you go down and then over two.” He explored that one here:

It was a wonderful first day back! My students and I really enjoyed the choral count (although they all spelled it coral:)! It was a very safe feeling knowing they were all saying it together, a bit different than the counting around the class.

-Kristin

MathMinds

by Kristin Gray.

Category Archives: Decimals

5th Grade: Decimal Place Value

Decimal Addition Card Sort

Fraction & Decimal Number Lines

Remainders: Division & The School Year

Creating Contexts for Decimal Operations

Multiplying Decimals Less Than 1 Whole

Making Decimal Predictions

Investigating Patterns

Connecting Whole Number Operations to Decimal Operations

Choral Counting – Decimals