Tag Archives: Math

Leveraging Digital Tools for Problem Posing

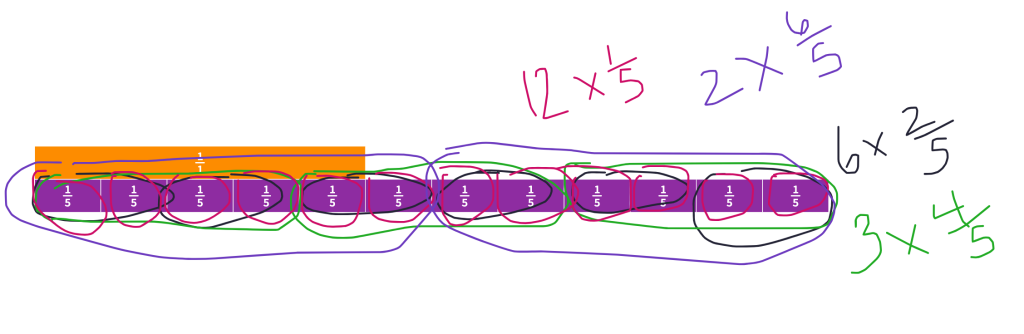
I have blogged a few times about problem posing using print materials and lately I’ve become really interested and excited about the potential for digital tools in this work!

If you are new to problem posing, below are a few slides from Jinfa and my NCSM presentation for background – each image is linked to an associated research paper.

What is problem posing?

Many activities can easily be adapted to provide opportunities for problem posing by removing task questions (left) and replacing it with different prompt options (right).

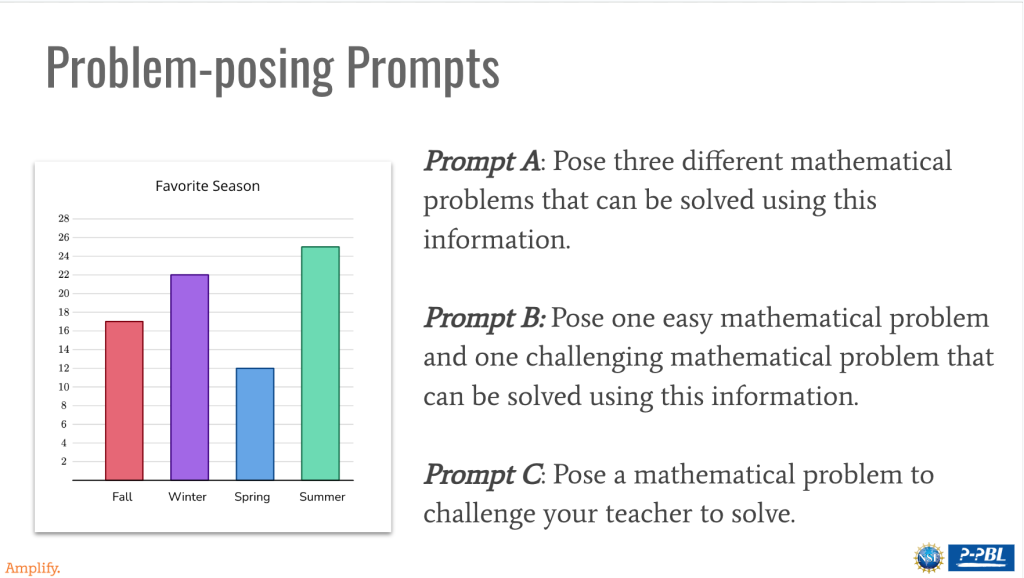
How can digital tools enhance problem-posing experiences?

Being relatively new to both problem posing and digital lessons, I have learned so much trying things out in math classes this year. As always, the more I learn, the more questions/ideas I have. Below are two digital lessons that involve different flavors of problem posing.

Lesson 1: Our Curious Classroom

You can click through the lesson screens to see the full flow, but in a nutshell, students answer questions about themselves and explore different data displays.

After answering the first survey question, we asked students for problems they could answer about their class data and recorded their responses (sorry for the blurry image, I had to screenshot from a video clip:).

The students then worked at their table to answer the questions based on their choice of display.

The lesson continues with more survey questions, data display exploration, and ends with students personalizing their own curioso character (see bottom of post for unrelated, cute idea).

Things I learned:

Student responses can be collected and displayed so quickly with digital which saved us more instructional time for posing and solving problems.
The capability to see data displays dynamically change from one to another enhanced the discussion about which display was most helpful to answer the problems and why.
Students were so motivated to answer questions about themselves, learn about their classmates (audio clip below), and ask and answer questions about their own class, not a fictitious one.

“What did you like about the lesson?”

Things I wonder:

While having the teacher record the questions on the board worked perfectly, I wonder if or how younger students might digitally input their own questions w/o wearing headphones for voice to text or having spelling errors that are challenging for others to interpret? Maybe something like a bank of refrigerator magnets to choose from?
During the lesson, could the teacher input student questions onto cards in the Card Sort in Desmos so they could then sort the problems based on structure before solving?

Lesson 2: Puppy Pile

In this lesson, students generate a class collection of animals, are introduced to scaled bar graphs, and create scaled bar graphs. This one has a different problem-posing structure than the the first lesson which was interesting!

In this lesson, students use the Challenge Creator feature. In order to pose their problem to the class, students create their own set of animals (left) and then select a scale and create a bar graph (right).

After submitting their challenge, students then pick up one another’s problems and solve them.

Things I learned:

Students were extremely motivated to create their own problems and solve the problems of others.
This version of problem posing allowed students to have more control over the situation around which they were formulating problems, which they really enjoyed.
Challenge Creator is an amazing tool for repeated practice that is MUCH more engaging than a worksheet of problems.

Things I wonder:

How could this activity structure support or extend the problem posing experience in Lesson 1?
What other K-5 math concepts would be great candidates for a Challenge Creator problem-posing activity?

Final thoughts

I think problem posing is such an important instructional structure whether done in print, digital, or a hybrid of the two. It is important, however, to also consider the math, student motivation, and amount of time students spend engaging in the problem-posing process when choosing the format we use.

I would love to hear about what you try, learn, and wonder whether you try these lessons or adapt other lessons for problem posing!

Unrelated by Adorable Idea…

After Lesson 1, Katie printed out their personalized Curiosos for the wall;)

Small Change, Big Thinking

Fraction Activity

In this 4th grade activity, students were writing equivalent multiplication equations for a fraction multiplied by a whole number and then discussing the relationship between the different equations. The curriculum activity was good and definitely addressed the learning goal, but there was definitely an opportunity to open it up for more student reasoning and ownership. For example, in its current form, students don’t have the chance to think about which whole numbers would work in their equations or play around with the properties.

Small Change

Adapting doesn’t always require huge lifts. For this activity, all we decided to do was change the prompt to 12/5 = ____ x _____ and ask them to find as many ways as they could to make the equation true. I got so wrapped up in their work and discussions, that I didn’t snag any pictures of that part of the lesson, but after they finished we pulled up polypad and asked them how we could show why they are are all equivalent using the fraction bars. We wanted to be sure they just weren’t proceduralizing it at this point of the unit, so pulling up the fraction bars felt like a nice grounding of the concept. The board looked like this before we erased to make space to circle the other expressions.

Making Connections

At this point, they couldn’t get enough and asked for another fraction to try, so we gave them 16/3. We saw so much great thinking and use of the commutative property when finding the whole number and numerator.

Their excitement alone was the first indicator that allowing more space for their choices was a great idea! And then, as I was walked around, a couple students asked if they could write division equations. Of course I said yes and walked away.

I came back to #7 and #8 on this board:

When I asked how she came up with those equations, she said she used her multiplication equations because multiplication and division are related. I left her with the question of how she might show that division on the fraction bars and class wrapped up. I can’t wait to check back in with her tomorrow to see what she came up with!

Next time you plan a math lesson, I encourage you to think about small tweaks you can make to open it up for more student voice, ownership, and opportunities to think big! And I don’t know if anyone is even talking much about math planning on Twitter (X) anymore, but if you are, I would love to think together about tweaking math activities. So, send some activity pics my way @MathMinds and we can flex our curiosity and creativity muscles in planning together.

-Kristin

Area and Perimeter of Squares – Student Noticings

11 Replies

This will be a quick post because I have a student-posed math problem that I need some time to reason through!

Today, students found the area and perimeter of squares that increase in side length by one each time. Students used a variety of models when building their squares from Minecraft carpets, to Geoboards to graph paper. Here is the completed activity sheet from their work: I then gave them a few minutes to talk to their tablemates about things they notice in their work. Here are the answers they shared as a class and I recorded on the board:

“An even dimension by even dimension = an even area”

“An odd dimension by odd dimension = an odd area”

“The perimeter goes up by 4 every time the square gets bigger”

“The areas are square numbers.”

“The areas go up by odd skip counting: +3, +5, +7…”
I was pretty excited because they really pulled out some great noticings and my next step was for them to choose one and find out why that was happening.

AND THEN THIS HAPPENED…

WOW, what a noticing!

Each pair of students chose one noticing from the board and worked on figuring out why that was happening. I had groups share the even dimensions = even area and perimeter going up by four. The tables that chose area going up by “odd skip counting” and the last one, left with no answer but excited to keep trying to “figure it out.”

Now, if you know why this last one works, please let me know that you know, but keep it a secret from me for right now! I want to sit and work through this one but I also need to know who to run to if I don’t get it!

I have found that you have to add the odd dimension area to the even dimension perimeter and if you do it the other way, it does not work. Why in the world does this work every time?

Had to share because it was such great conversation and I left having the hunger to sit and work thru the math….better yet, the students did too.

Enjoy and please let me know if you know why that is working because I may be reaching out!!

-Kristin

**Follow up comment: Thanks to my Twitter buddies, I worked my way to the visual of this problem. It was much easier to make sense of this algebraically, but the “why” took a lot of square drawings and scribbles! It was hard to make the connection between perimeter being the distance around to it being one side or a square tile. Here is part of my working on my Geoboard app…

So the area of a 1×1 + the perimeter of a 2×2 = the area of a 3×3.

Always, Sometimes, Never – Quadrilaterals

5 Replies

One of my “go to” questions for students when they are working through math in my classroom is, “Will that always be true?” I find it pushes the thinking to another level where students are looking for examples and/or non-examples.

On Twitter one evening I found a blog post by @lisabej_manitou that was the embodiment of my go-to question: http://crazymathteacherlady.wordpress.com/2013/11/20/always-sometimes-never/ . Can you say perfect timing, as my class is in the midst of quadrilateral properties/classifications?

I gave each group of students Lisa’s sheet, clarified any key vocabulary questions and the conversations started rolling!

We have been doing a lot of work with classifications and discussing all of the classifications polygons can have, but this activity took that to a great new level. The “Sometimes” column has to be my favorite because it requires to think of both cases, true and not true.

One group had a very “heated” debate on the “Square is a Rectangle” card, which if you asked me ahead of time, would not have been the one I expected to hear such debate (at least in the respect that it was). I know that often students come into this unit having formed or memorized some form of the statement that “Squares are rectangles, but rectangles aren’t squares.” Whether it is taught or formed on their own, it is put to test when faced with the always, sometimes, never. Those are the words that are key in the misconceptions built around that statement. That was the conversation I expected to hear when I walked over to the group and looked at the card in question…however it was actually quite different reasoning!

One member of the group was literally “Starting to sweat” (her words) from this conversation. She was trying to explain to her group why a square is SOMETIMES a rectangle. Her reasoning was this: (I had to have her write it down so I could use it later in class and she needed a breathing moment away from her group)

She made an interesting point for students to reason about. If a rhombus can be a square, and rhombuses (or is it rhombi?) are not rectangles, squares can’t always be rectangles.

I pulled the class together to discuss this point because there were others agreeing with her reasoning. They SO wanted me to tell them who was right and who was wrong….um no way! I asked them what would prove or disprove this argument to them. One group said they would need her to show them an example of when a square was not a rectangle because if it is sometimes, it has to be a case of when it is and isn’t.

And, class dismissed. They left wanting to continue: creating arguments, critiquing the reasoning of other, making mathematical models, looking for patterns in their reasoning….I would say it was a great day in math!

I am SO glad they didn’t finish yet bc I am planning on recording some conversations on Monday to post.

Thanks Lisa for the great lesson!

-Kristin

Math Workshop

Rethinking Homework Pt 2

1 Reply

For those interested in the follow-up on my homework change after reading my first post: https://mathmindsblog.wordpress.com/2013/12/28/rethinking-homework/ , this is my reflection on the the process…

1 – Choosing the problems – I felt a bit overwhelmed with choosing the problems to put on the homework page. Who would have thought? It is just two problems, right? Unlike the “typical” homework that goes along with what we did in class that particular day, I wanted to use it more to see if students truly learned the concepts we worked with over the course of the first half of the year. With that, I chose to use a volume problem from Illustrative Math: http://www.illustrativemathematics.org/illustrations/1308 , which was our first unit of the year, on one side and on the opposite side I put the addition problem: 3 3/4 + 5 1/8, and asked students to show two different ways to solve the problem. I thought one problem in context along with one problem without context was a nice mix.

2 – Students showed great responsibility – I purposely gave the paper to the students on Thursday and assigned it to be due the following Monday so students had two nights to actually get the paper home (sometimes that is the hardest part:) and then two more nights to find an adult. I was very excited to get 98% of the papers back from the students and they seemed really excited to tell me about what their parents had said about their work. I heard everything from “They said they wouldn’t solve it that way” to “They were so proud of my vocabulary.” Loved it!

3 –Parent response – I had great parent response both on the sheet and in conversations after the fact. Here are just a few examples of comments made….

I loved that I got praise, concerns as well as strategies! It was pretty awesome and hopefully led to some great conversations at home!

4 – My response – I wanted to take time to look through the work and plan my next steps but I also wanted to acknowledge the parents comments and/or concerns right away. That day, I sent a quick email to the parents to first thank them for taking the time to work with their child on math and to also let them know that I saw their questions/concerns and that although they may not get a direct response from me on each individual comment, I will be working with their child based on their work.

5 – My plan from here – I have math workshop planned for tomorrow in order to give me time to work with the students who may have misconceptions or maybe just not using an efficient strategy in their work with volume and/or fractions. I found the fraction work pretty amazing and, I cannot lie, it really made me feel great about the work we had just done in our fraction unit. They really used some creative thinking in looking for a second strategy!

Overall, I completely loved the homework and the result! I am in the process of creating my next one to give the students tomorrow. I don’t think I will make any changes at this point in the layout of the sheet at this point. I do think that when I give a problem with no context next time, I will ask the students to write a story to go along with it. I did find that more struggled with the volume question, possibly because of the context, so I really want to work on the students moving in and out of context in mathematics.

– Kristin

Student samples in case you are interested:

Rethinking Homework…

8 Replies

One of the things on my vacation “To-Do” list is to rethink the way I do homework in my classroom. The concept of homework is something I constantly go back and forth with in my head, trying to find the perfect balance of meaningfulness for my students.

Some things I have learned, loathed, and/or questioned about homework…

More problems does not equate to more meaningful. If a student can do 2 problems correctly, chances are they can do 20. Conversely, if they can’t do 2, 20 will be extremely painful.

Some parents will want more homework, some will want less. This is not necessarily based on any particular demographic, it is varying. I find this more stems from parents reflecting on their own school experience and homework load. What they believe makes students “better math students.”

Some students will have help (resources) at home and others will not. Then I end up grading/giving feedback on parent’s work or work that has been corrected by the parents vs the students that did it completely alone. Not equitable in my eyes.

Some will never remember to bring their homework home and if they do, will lose it on the way back to school. So, then I feel like I am grading a student on responsibility and not their math understanding.

Homework should be meaningful, however what is meaningful varies from student to student. How can I make it meaningful for the 45 individuals in my math classes?

Homework can be beneficial to involve parents in what is being learned in class. I do newsletters and parent math nights, but really homework seems more accessible. I want students talking math their parents and questions that may arise from parents. Homework can open those doors, but I don’t want it opened in a negative “my child couldn’t do this and I had to show them how I solve it” kind of way.

Am I doing the students a disservice if they hit 6th grade and beyond and the homework load increases dramatically on them? Am I responsible for “preparing” them for what will happen in future grades? (whether it be best practice or not).

I feel there is benefits to students being held accountable for completing assignments outside of school. From time management to the organization and responsibility of completing a task are life skills that I feel are extremely important. But, how do grade/give feedback to those skills? If it doesn’t somehow “count,” some students will not really have the motivation to do it.

I feel like the time spent grading/giving feedback on homework could be better utilized in planning upcoming lessons.

This list could go on and on, but for the sake of time and actually being able to check something (actually two things bc blogging was also on there) off my to-do list, here is what I have come up with thus far…

I will assign one or two problems for students to solve and explain their reasoning. I will attach those problems to applicable standard(s) (including the Math Practices) so parents who would like, can see why certain things are being done in class. I will have it due over a few day period so students can manage their time and organization. Hence leading to the first part of the page:

Now the second part is hard because I am trying to break a mindset. I want the students to explain their reasoning to the problem and parents (or any adult) to check off whether the student could explain it clearly or not. NOT FIX IT! Then I left a box for any questions or concerns for me… second part:

I am hoping this offers as more of a formative assessment for me that connects parents to the learning in our classroom. For the students who do not always have parents available, any adult will do…this way the students could use one of their other teachers in school that they see each day.

I am also hoping that by having students explain their reasoning to someone other than me (because most times I “get where they are coming from mathematically”) it will force them to be accurate and clear in their explanations, putting a big emphasis on the Math Practices.

I am hoping this opens more communication between the parents who I may not have reached the first half of the year.

I am hoping this gives me more time to focus on planning and instruction time than grading or going over homework assignments.

As always, a work in progress. Any thoughts are appreciated!

Now, time to check two things off my list…for now..

-Kristin

Brainstorming with Minecraft…

1 Reply

Like many teachers right now, I am starting my Winter Break “To Do List.” One of the things at the top of my list is planning for our upcoming math unit. Since we have recently received our iPads, I am constantly trying to find a way to use them as a learning tool in my classroom. I don’t want them to just be a “paper replacement” but instead a part of the learning process. Our upcoming unit is Measuring Polygons which includes work with area and perimeter and as I read Fawn’s AMAZING Hotel Snap task the other day, I got inspired!

In case you haven’t seen it: http://fawnnguyen.com/2013/12/10/20131027.aspx

I love SO many things about this task: the collaboration, the challenge, the math, the Math Practice reflection, all materials to go along with it, accessibility for all students…and I could go on and on….it is awesome!

For my 5th graders, I am going to try to recreate this task using Minecraft, since the app is on their ipads and they are just dying to use it in class. I think it has such potential for some seriously amazing math and creations…not to mention the engagement factors of a competition and Minecraft…they will be in math heaven!

I am in the beginning planning stages, but to keep my thoughts organized (and get some feedback), I figured I would just start typing my initial thoughts and/or questions I am having…

1 – To start, our next Social Studies unit of study is Economics so I had the students price out the materials in Minecraft today. It was great conversation of durability and availability. If a material was difficult to “find” or “craft” in the game, the higher the prices. Here is an example of a piece of the students’ work:

I plan on creating a spreadsheet in Numbers for them to keep track of their block usage during construction.

2 – I am thinking they will build a resort instead of a hotel because of the options to put in sidewalks, pools, petting zoos and such to increase revenue and include volume into the equation. Question: How do I factor in profit of having extra amenities? Does a bigger pool bring in more money? How in the world do I price a petting zoo (because they so want to put animals in there)?

3 – The rooms of the resort will still follow the window guidelines in Fawn’s original task, but the rooms will be 3-D so I am going to allow them to put beds in each room. That would change the pricing of the rooms not only by window, but by accommodations (single vs double vs king). Question: Will the bed and window pricing be overwhelming and time consuming and take away from the challenge of finding most profit? Should I make it one or the other?

4 – Love the scoring, keeping that exactly the same.

5 – Question: Do I give them a block limit or spending budget? Is there an advantage of seeing who can make the most money with the same number of cubes vs who can make the most money with the same budget?

6 – I would like to incorporate area and perimeter relationships here so I am thinking it has to be a “gated” resort. Possibly: What would happen to you cost of gating if you doubled the area of your resort? or How could you arrange the resort to keep the area you would like but keep your gating cost the lowest?

I would first like to thank Fawn for the inspiration and amazing resources! I would love any and all thoughts additions/deletions on the task. I always have my cubes bagged and ready for use if this is a bust!

Thanks!

Kristin

You Never Know What They Know Until You Push Their Thinking….

To PD or Not PD..That Is the Question

3 Replies

The past two years as Math Specialist, I was in a position in which I was continually planning and attending Professional Development on a regular basis. I am a learner, so I frequently got frustrated and a bit upset when teachers complained about attending the PD. I would hear such things as, “I need time to grade my papers” or “Sub plans are such a pain to write.” How could they not love these learning experiences as much as me?

Fast forward to this year, I am back into the classroom, and I completely feel their frustrations. I have papers that need to be graded, I despise sub plans, and most importantly, l have lesson plans that I need time to think about & dig deeper into. Time, as always, is a high commodity. So, as I was in my classroom Thursday evening, writing sub plans (or more accurately procrastinating by finding anything in my classroom that needed to be done BESIDES writing the plans) I found myself thinking that it would be so much easier to not attend the PD (it was by choice I was going) and just stay in the classroom on Friday. No sub plans, and I would have my planning time to get the paper grading and lesson planning done.

This was it, this is the point where teachers (me included) need to step out of their immediate surroundings, look at the bigger picture, and ask themselves the following questions….

1. How can I continue to improve student learning in my classroom if I don’t dig deeper into my content area(s)?

2. How can I grow as an educator alone?

3. How can reflecting on my own teaching with others improve my classroom experiences?

4. How can what I know about teaching help others in my network?

5. There is always SO much more to learn. Not a question, I know, but it is my driving force as an educator.

And….How great is it to have breakfast and lunch made for me and I can use the bathroom anytime I want 🙂

Needless to say, I always try to attend professional development when offered the opportunity and after leaving my PD on Friday I just found myself smiling. I love talking to others with the same passion for mathematics and teaching as myself. I learn so much and just flat out have fun while talking about impactful issues in education. We all want what is best for our students and staff and work together to make great things happen.

Don’t get me wrong, I am picky when choosing my PD. It must be relevant. I have sat in a mandatory PD or two (hundred) that have not been what I needed, but I try to find at least one thing I can take away. Even through the bad experiences, I grow. If a presenter is not engaging, I think about what I can do when I facilitate to be engaging. If the content is confusing, I think about how I can clarify things when I facilitate a professional development. I don’t let one bad experience kill all professional development opportunities for me. They are independent variables, like a die. One roll does not impact the next. One bad professional development does not impact the next one.

In the end, I owe it to my students to go. If I am learning more, they will be learning more.

Happy Saturday,

Kristin