Some things I am wondering right now about 3rd grade multiplication…
- When students notice 4 x 3 is the same product as 3 x 4 and say, “The order doesn’t matter,” how do you answer that question?
- Is there a convention for writing 4 groups of 3 as 4 x 3?
- Is there a time, like when moving into division or fraction multiplication and division when the order does matter in solving or in thinking about the context?
Answers I have right now for these questions….
- Right now, since they are just learning multiplication, I ask them what they think and why.
- I think there is a bit of a convention in my mind because the picture changes. Three baskets with 2 apples in each is different than 2 baskets with 3 apples in each. Also, when reading the CCSS it seems that way.
- I am still thinking about division but it makes me think that this would be the difference between partitive and quotative division. I also think when students begin 4th fraction multiplication, they are relating it to what they know about whole number operations, so 4 x 1/2 is 4 groups of 1/2. This seems important.
The 3rd grade teachers and I have been having a lot of conversation about these ideas. The students have been doing a lot of dot images and some feel strongly that the two expressions mean the same thing because they can regroup the dots to match both expressions. Others think they are different because the picture changes. All of this seems great, but then students are taking this reasoning to story problems. For example, given a problem such as, There are 5 shelves with 6 pumpkins on each shelf. How many pumpkins are on the shelves? students will represent that as 5×6 or 6×5. Is that a problem for me, not really if they have a way to get the 30, but should it be? I am not sure.
I went into a 3rd grade classroom to try some stuff out. I told them I was going to tell them two stories and wanted them to draw a picture to represent the story (not an art class picture, a math picture) along with a multiplication equation that matched.
1st story: On a grocery store wall there are 5 shelves. There are 6 pumpkins on each shelf.
2nd story: On another wall there are 6 shelves with 5 pumpkins on each shelf.
I asked them if the stories were the same and we, as I anticipated, got into the conversation about 5×6 vs 6×5 and what it meant in terms of the story. They talked about 5 groups of 6, related the switching of factors to addition and then some talked about 6 rows of 5.

From this work, many interesting things emerged…
- Some students had different answers for the two problems. They obviously did not see the two expressions as the same because they struggled with 5 groups of 6 as they tried to count by 6’s and forgot a row.
- One student said they liked the second problem better because she could count by 5’s easier than by 6’s.
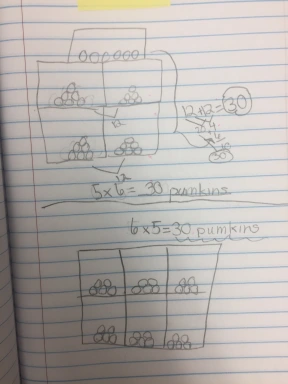
- Students skip counted by 5’s but added 6’s when finding the 5 groups of 6.
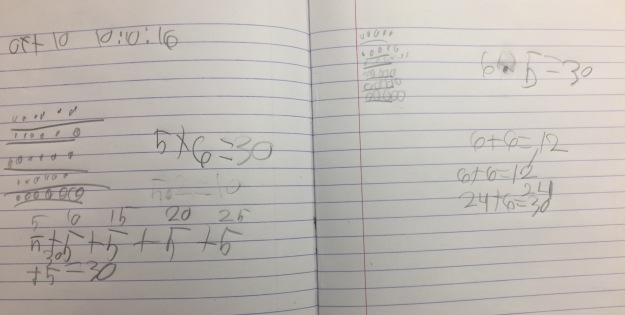
- One student noticed the difference between 5 and 6 and could relate that removing one shelf was just adding a pumpkin to each of the other rows.
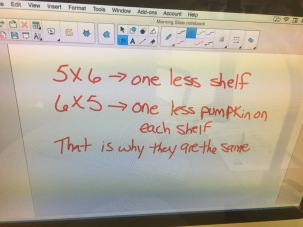
- One student showed how he used what he knew about one to switch the factors to make it easier to solve.
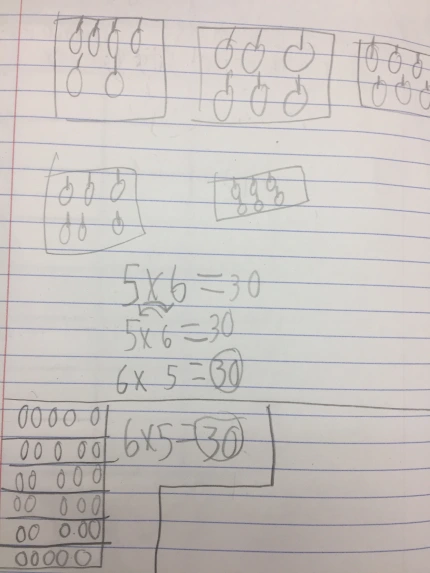
But they keep asking Which one is right? and I tell them I don’t have an answer for them. I just keep asking them:
Is the answer the same?
Is the picture the same when you hear the story?
After chatting with Michael Pershan yesterday, I am still in a weird place with my thinking on this and I think he and I are in semi-agreement on a few things (correct me if I am wrong Michael) …Yes, I think “groups of” is important to the context of a story. I want students to know they can find the answer to these types of problems by multiplying. I want students to be able to abstract the expression and change the order of the factors if they know it will make it easier to solve BUT what I cannot come to a clear decision on is…
If we should encourage (or want) students to represent a problem in a way that matches the context AND if the answer is yes, then is that way: a groups of b is a x b?


You have asked and thought through some good questions. I won’t be much help with the nitty gritty of them, but maybe something here will resonate as your post took my thoughts up a few levels .
Conventions, things that are generally agreed upon, exist in math. In a classroom where students are engaged in sense-making, conventions may become more apparent (e.g. “hmm, I can’t seem to reason out why I’m using this notation, name, or format”.) This is more challenging in a classroom where rote learning rules the day and everything looks arbitrary.
But even in a classroom where sense-making rules the day and students are regularly engaged in mathematical practices, the need for conventions may not be apparent right away or even for some time. You allude to this with your questions. When (and does) 3 x 4 vs. 4 x 3 become important?
My question is, over time, can we help students learn how to answer this question themselves?
Maybe not in this classroom, or with these students, but I wonder if there’s an opportunity to play the long-game. What I mean is, when a student asks “does the order matter?” then how can teachers engineer ways for them to explore if it matters based on what they currently know. And then, have a conversation afterwards, something along the lines of “do you think this will always be true?” What might you learn in the future where you will need to ask yourself “hmm, does it matter if I use a*b or b*a here?”
I think about how students sometimes feel duped because they learned a half-truth at a younger age only to find out it is wrong or superseded as they get older.
This is probably akin to the Mr. Miyagi method of teaching. The seeds are planted, the practices are learned, but some of the most important lessons don’t happen that day. They are learned at some undetermined time in the future when enough water and sun have made that seed fertile for the student.
I wonder what learning like this may do for our students’ sense of themselves as mathematicians, as learners, as people with agency. I may be too pie in the sky for the very real challenges you’re grappling with right now at the classroom level but I’m glad to have this post to think about them.
LikeLiked by 1 person
So many great questions here. All of this really does matter when we’re talking about having a deep understanding of fundamental mathematical ideas like the commutative property of multiplication. I can address a few of your questions, though. Saying 4 x 3 is “4 groups of 3” is, indeed, a convention used in the U.S. and other parts of the world, but not everywhere. Some places say something like 4×3 means “4, three times” –so the opposite of what we say here. I like the convention we use because I find using the “groups of” language for multiplication to be more helpful to students than just saying “times.” When I work with teachers, I make a big deal about order because I want them to use language and draw pictures in ways that really helps kids see the connection between the mathematical symbols and the context/pictures.
When kids are struggling with the idea that 4 groups of 3 DOES look different than 3 groups of 4 when in context, and yet we still say 4×3=3×4, they are developing an important understanding of what the “=” actually means in math. It does not mean “is exactly the SAME as” but rather “has the same VALUE as.” This is a subtle, but important, difference. In math, a really powerful tool that can be used in problem solving is that expressions that have the same VALUE can be used interchangeably, even if they look different. Your student who said she “liked the second problem better because she could count by 5’s easier than by 6’s” is onto something really important mathematically–when she wants to find the answer to a x b, she can choose to solve the problem b x a if it’s easier for her, because they have the same value! Students can’t really make sense of why this is true unless you give them lots of chances to talk about why a groups of b will always have the same value as b groups of a. Honestly, the questions you’re asking and the work you’re showing here from kids really seems to be giving them just that opportunity. The only other questions I might ask of a student who writes “3×4” for “4 groups of 3” would be, “Tell me about what this expression 3×4 means to you and how it relates to the problem.” I wouldn’t insist on them writing it in the “right” order, because order is a convention, but I would want to know that they understand what the expression means.
LikeLiked by 2 people
I love that one of your students realized that removing one shelf was just adding a pumpkin to each of the other rows! I see the question you pose 2 ways: (1) yes, the order does matter in how they represent the multiplication expression because as their illustrations show, 5×6 is different that 6×5 when it comes to the situations described in the word problem. But (2) if we want our students to understand the commutative property of multiplication, they have to understand that the 2 expressions are equal and order doesn’t matter. Sorry to throw out another question, but is ‘equal’ the same thing as ‘the same’?
LikeLike
We really aren’t that precise in our language. When we say 3 groups of four, we’re still wallowing in abstract. Still, if I think of ten examples of “3 groups of four fish”… it’s the ‘container’ that’s coming first and the ‘amount in the container’ that’s coming second.
The big wild card is different people are going to sense and see the issues differently, too.
LikeLike
It is so lovely that you are taking time to explore multiplication, the commutative property, and what it means for expressions to be equal. This is so much richer than asking “How many pumpkins?” Your activity and questions send a strong message about how much your value student sense-making (as opposed to only valuing the “right” answer).
Honestly, I was not aware that “a x b” conventionally meant “a groups of b”; what may matter more is asking a student about her reasoning. For example, 6 (pumpkins per shelf) x 5 (shelves) and 6 (shelves) x 5 (pumpkins per shelf) both might be written as 6 x 5. The labels are key.
LikeLike
Makes me think of Graham’s progression videos when he said that the context dictates the representation (not exact words).
This also makes me think of SMP 2. Students have to pull the math out of a context, work on the math, and then put the math back into the context. So I would think that the order does matter. I think it’s great to understand the commutative property of multiplication, but if you’re attending to SMP 2 and SMP6 I think the order does matter. Thoughts?
LikeLike
This issue has been raised with much heat and less light on YouTube, where people who are convinced that anything that is in the Common Core is the work of Satan. Allegedly, there was an exam (try pinning down WHERE this exam took place, who wrote it, who gave it, etc., and you’ll have a hard time getting responses that are on point, let alone definitive information), in which primary grade students were asked a question involving multiplying 5 * 3 (or perhaps it was 3 * 5) and asked to show, explain, or represent their answer using addition, and students who wrote 3 + 3 + 3 + 3 + 3 were marked wrong, or perhaps it was those that wrote 5 + 5 + 5 were marked wrong.
The point is that in classic “parents with pitchforks” fashion, comments made clear that this was the start of the fall of the American Empire writ large before our eyes.
Frankly, if CCSS-M or teachers in early grades are teaching that there is a specific way that kids MUST represent a product of two numbers as addition (and I’m of two minds on that notion to begin with), I’m troubled. Clearly, a 5 x 3 array is the same as a 3 x 5 array; if you don’t believe me, rotate the paper you’ve drawn the array on 90 degrees in either direction. One of the beautiful things about the area/array model of multiplication is that it shows rather clearly without any formal proof that multiplication of whole numbers is commutative (not a word I’m likely to use when speaking with kids in early grades, but certainly one I expect their teachers to know and understand). And they should already know (both the kids and the teachers) that this same thing is true for addition. So if we’re going to insist that multiplication is* repeated addition (and I beg you never to put it precisely that way), we certainly are going to have a hard time getting away from the commutative properties of addition and multiplication of whole numbers (and later, of integers, rational numbers, and, much later, real numbers and complex numbers). And we should be prepared to think long and hard about these things as teachers and to spend more than a few minutes of class time on it. A lot more. Because some day, many of those students are going to discover that multiplication isn’t always commutative (for example, the product of two square matrices, A and B, will, in general, NOT equal the product of B and A; when you study the definition of matrix multiplication, and if you’re lucky enough to explore what matrix multiplication is about on a more abstract level, you’ll start to understand precisely why it should NOT be commutative).
But getting back to the link between addition and multiplication, it’s a tough sell to students and to me that if you ask for a representation of 3 x 5 without stipulating that one of those represents the number of groups and one represents the size (cardinality) of each group that it’s wrong to write it as 5 + 5 + 5 or that it’s wrong to write it as 3 + 3 + 3 + 3 + 3, and I say that being fully cognizant of the fact that there’s a world of difference between, say, a job that takes 3 people working 5 hours each and one that takes 5 people working 3 hours each if you only have 3 people to do the job or absolutely need to have it done in 3 hours. It’s still a 15 person/hour job, but you can’t yell that at the job if your available workers or your time limitations demand one thing or the other.
The problem here is that the real world is the real world; mathematics entails abstract thinking, and even elementary arithmetic gets abstract pretty quickly. We try these days to make it easier for kids by keeping everything as concrete and hands-on as possible, but even there we get betrayed. 3 x 5 = 5 x 3 and 5 + 5 + 5 = 3 + 3 + 3 + 3 + 3, and I don’t particularly care if some convention insists that one of those factors in the product is the multiplier and the other is the multiplicand because, frankly, like subtrahend and minuend, these are words that no one outside of K-5 is likely to ever use or hear uttered. And it goes against common sense to make this particular molehill into a mountain, as far as I can see.
*For why I want teachers and everyone to rethink “Multiplication IS Repeated Addition” [MIRA] as opposed to the notion that you can get the answer to a multiplication problem through the process of doing repeated addition (though you might want to think about how you would calculate pi times the square root of 2 that way), see a series of articles by Stanford mathematician Keith Devlin, starting here: http://www.maa.org/external_archive/devlin/devlin_06_08.html
And here’s one of my own blog pieces on the same controversy: http://rationalmathed.blogspot.com/2010/02/keith-devlin-extended.html
Finally, I love the fact that you are raising these issues. More teachers should be.
LikeLiked by 1 person
Howardat58: Sweet! Now I’m convinced. 🙂
LikeLike
Updating the above comment, people interested in the MIRA controversy might find my recent interview with Keith Devlin of merit: https://mathblog.com/keith-devlin-ambassador-mathematics-many-eras/
LikeLike
@Howardat58: “Common Core writes”? What do you mean?
LikeLike
@Howardat58: you mean that those things are sold that way?
I’ve always had some confusion with what people mean (particularly people who themselves may not know what they really mean) when they say things like “50% more” – or “twice as much.” In my mind, the first means 1.5 times the original amount; the second means 100% more than the original amount or 200% of the original amount. And those last two represent the same quantities but not the same way of getting there. The first is additive, the second is multiplicative.
But I wouldn’t bet my life that I’m “right,” and I think these are areas where there is confusion between ordinary language and mathematical representation. The latter should be unambiguous, but the former? Not so much.
LikeLike
It’s great that you’re digging deeper into concepts and inspiring the children and others to do likewise!
Personally I believe that the children first need to be taught multiplication as you suggest ie that a x b is a sets of/groups of/rows of b. That way it can be illustrated better concretely and pictorially and is easier to visualise I believe.
(see the images here: https://sites.google.com/site/primarycpd/latest-news/multipleways)
Then when they’re comfortable with that I usually begin to introduce the commutative property or “turnaround facts” similar to this:
5 x 4 could be seen as 5 plates of 4 cookies which is 20 cookies
4 x 5 could be seen as 4 plates of 5 cookies which is still 20 cookies
So the answer is the same, but it looks differents ie “same value, different appearance”
I also get the students to use a 100 dots grid:
Again to see image click here: https://sites.google.com/site/primarycpd/latest-news/multipleways)
3 x 6 = 3 rows of 6
6 x 3 = 6 rows of 3
There are still 18 dots each time but each arrangement/array looks different.
However, if you rotate/turn the 100 dots around, the arrangements/arrays begin to look very similar, hence the phrase “turn-around facts” ie 6 x 3 = 3 x 6
The beauty of the 100 dots grid is that it can also be used to explore the distributive property of multiplication also i.e. that in multiplication we can break apart groups/arrays into smaller/easier/more familiar groups/arrays and then re-combine them to get an answer, ie
3 rows of 6 is clearly the same as 3 rows of 5 plus 3 rows of 1
3 x 6 = (3 x 5) + (3 x 1) = 15 + 3
Keep wondering and re-thinking!
LikeLike
Pingback: …equals (write answer here). | Just MSU
I just had this discussion the other day. I don’t know the answer either. Couldn’t a person make the argument that 6 x 5 works because they have 6, five times? In addition, do we worry about the order of the addends according to the context? I suppose that for standardized testing purposes, we should have a conventional method, but I don’t know that I believe that the order of factors (or addends) necessarily demonstrates student understanding.
LikeLike
It is a good discussion where I am myself been having this confusion, when there is an illustration such as described in original discussion by Kristin Gray, 5 shelves with 6 pumpkins each and 6 shelves with 5 pumpkins each, pictures and when written in groups of are ofcourse different, but writing in a mathematical sentence how is it different. As 5 shelves with 6 pumpkins each can be written as 5 x 6 is same as 6 shelves with 5 pumpkins each can be written as 6 x 5.
Can someone clarify this.
LikeLike
ofCe) AND 5078=1262 AND (4637=4637
LikeLike
ofCe’) AND 8608=8753 AND (‘QdSE’=’QdSE
LikeLike
ofCe’ AND 9338=7820 AND ‘PBcm’=’PBcm
LikeLike
Even though 4×3 is the same as 3×4, If we had to choose an order, I would say that
4 x 3 = 4+4+4 and NOT 4×3 = 3+3+3+3. Why you ask?…
Think about this…
If 4×3 = 4+4+4 = 12
then 4 pumpkins inside 3 shelves… equals 12 pumpkins… and it makes sense,
but if 4×3= 3+3+3+3 = 12
that would mean that 4 shelves inside 3 pumpkins equals 12 shelves!… and it doesn’t make sense.
Shelves can’t fit inside pumpkins, but pumpkins can fit inside shelves.
If we were to prioritize the shelves then that would be a division.
4 shelves filled with 3 pumpkins.
Another way of looking at it is…
4(apples) x 3 = 4(apples)+4(apples)+4(apples) = 12 apples!!
without assigning the “shelves” value to number 3.
There should be a comments editing option 😛
LikeLike